Rectified truncated icosahedron

Rectified truncated icosahedron
Schläfli symbol	rt{3,5}
Conway notation	atI[1]
Faces	92: 60 { }∨( ) 12 {5} 20 {6}
Edges	180
Vertices	90
Symmetry group	Ih, [5,3], (*532) order 120
Rotation group	I, [5,3]+, (532), order 60
Dual polyhedron	Rhombic enneacontahedron
Properties	convex
Net

The rectified truncated icosahedron is a polyhedron, constructed as a rectified truncated icosahedron. It has 92 faces: 60 isosceles triangles, 12 regular pentagons, and 20 regular hexagons.

Images

Dual

By Conway polyhedron notation, the dual polyhedron can be called a joined truncated icosahedron, but it is topologically equivalent to the rhombic enneacontahedron with all rhombic faces.

Related polyhedra

The rectified truncated icosahedron can be seen in sequence of rectification and truncation operations from the truncated icosahedron. Further truncation, and alternation operations creates two more polyhedra:

Name	Truncated icosahedron	Truncated truncated icosahedron	Rectified truncated icosahedron	Expanded truncated icosahedron	Truncated rectified truncated icosahedron	Snub rectified truncated icosahedron
Coxeter	tI[2]	ttI[3]	rtI	rrtI	trtI	srtI
Conway			atI[4]	etI[5]	btI[6]	stI[7]
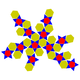
Image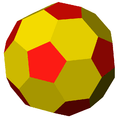
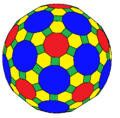Net
Conway	dtI = kD[8]	kdtI[9]	jtI[10]	otI[11]	mtI[12]	gtI[13]
Dual
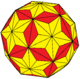Net

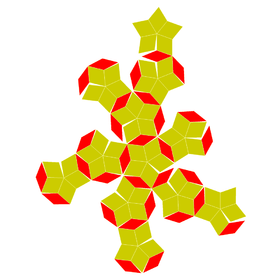See also

• Rectified truncated tetrahedron
• Rectified truncated octahedron
• Rectified truncated cube
• Rectified truncated dodecahedron

References

• Coxeter Regular Polytopes, Third edition, (1973), Dover edition, ISBN 0-486-61480-8 (pp. 145–154 Chapter 8: Truncation)
• John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5

External links

• George Hart's Conway interpreter: generates polyhedra in VRML, taking Conway notation as input