Largest known prime number
As of January 2016, the largest known prime number is 274,207,281 − 1, a number with 22,338,618 decimal digits. It was found in 2016 by the Great Internet Mersenne Prime Search (GIMPS).

Euclid proved that there is no largest prime number, and many mathematicians and hobbyists continue to search for large prime numbers.
Many of the largest known primes are Mersenne primes. As of November 2016, the six largest known primes are Mersenne primes, while the seventh is the largest known non-Mersenne prime.[1] The last 16 record primes were Mersenne primes.[2][3]
The fast Fourier transform implementation of the Lucas–Lehmer primality test for Mersenne numbers is fast compared to other known primality tests for other kinds of numbers.
The current record
The record is currently held by 274,207,281 − 1 with 22,338,618 digits, discovered by the GIMPS in 2016.[4] Its value is:
300376418084606182052986098359166050056875863030301484843941693345547723219067994296893655300772688320448214882399426831... (22,338,378 digits omitted) ...
717774014762912462113646879425801445107393100212927181629335931494239018213879217671164956287190498687010073391086436351
The first and last 120 digits are shown above.
Prizes
The Great Internet Mersenne Prime Search (GIMPS) currently offers a US $3000 research discovery award for participants who download and run their free software and whose computer discovers a new Mersenne prime having fewer than 100 million digits.
There are several prizes offered by the Electronic Frontier Foundation for record primes.[5] GIMPS is also coordinating its long-range search efforts for primes of 100 million digits and larger and will split the Electronic Frontier Foundation's US $150,000 prize with a winning participant.
The record passed one million digits in 1999, earning a $50,000 prize.[6] In 2008 the record passed ten million digits, earning a $100,000 prize and a Cooperative Computing Award from the Electronic Frontier Foundation.[5] Time called it the 29th top invention of 2008.[7] Additional prizes are being offered for the first prime number found with at least one hundred million digits and the first with at least one billion digits.[5] Both the $50,000 and the $100,000 prizes were won by participation in GIMPS.
History
The following table lists the progression of the largest known prime number in ascending order.[2] Here Mn= 2n − 1 is the Mersenne number with exponent n. The longest record-holder known was M19 = 524,287, which was the largest known prime for 144 years. Almost no records are known before 1456.
| Number | Decimal expansion (only for numbers < 1050) |
Digits | Year found | Notes (for larger Mersenne primes, see Mersenne prime) |
|---|---|---|---|---|
| 11 | 11 | 2 | ~1650 BCE | ancient Egyptians (disputed)[8] |
| 7 | 7 | 1 | ~400 BCE | It was known to Philolaus that 7 is a prime[9] |
| 127 | 127 | 3 | ~300 BCE | It was known to Euclid that 127 and 89 are primes[10][11] |
| M13 | 8,191 | 4 | 1456 | Anonymous discovery |
| M17 | 131,071 | 6 | 1460 | Anonymous discovery |
| M19 | 524,287 | 6 | 1588 | Found by Pietro Cataldi |
| 6,700,417 | 7 | 1732 | Found by Leonhard Euler | |
| M31 | 2,147,483,647 | 10 | 1772 | Found by Leonhard Euler |
| 67,280,421,310,721 | 14 | 1855 | Found by Thomas Clausen | |
| M127 | 170,141,183,460,469,231,731,687,303,715,884,105,727 | 39 | 1876 | Found by Édouard Lucas |
| 20,988,936,657,440,586,486,151,264,256,610,222,593,863,921 | 44 | 1951 | Found by Aimé Ferrier; the largest record not set by computer. | |
| 180×(M127)2+1 | 79 | 1951 | Using Cambridge's EDSAC computer | |
| M521 | 157 | 1952 | ||
| M607 | 183 | 1952 | ||
| M1279 | 386 | 1952 | ||
| M2203 | 664 | 1952 | ||
| M2281 | 687 | 1952 | ||
| M3217 | 969 | 1957 | ||
| M4423 | 1,332 | 1961 | ||
| M9689 | 2,917 | 1963 | ||
| M9941 | 2,993 | 1963 | ||
| M11213 | 3,376 | 1963 | ||
| M19937 | 6,002 | 1971 | ||
| M21701 | 6,533 | 1978 | ||
| M23209 | 6,987 | 1979 | ||
| M44497 | 13,395 | 1979 | ||
| M86243 | 25,962 | 1982 | ||
| M132049 | 39,751 | 1983 | ||
| M216091 | 65,050 | 1985 | ||
| 391581×2216193−1 | 65,087 | 1989 | ||
| M756839 | 227,832 | 1992 | ||
| M859433 | 258,716 | 1994 | ||
| M1257787 | 378,632 | 1996 | ||
| M1398269 | 420,921 | 1996 | ||
| M2976221 | 895,932 | 1997 | ||
| M3021377 | 909,526 | 1998 | ||
| M6972593 | 2,098,960 | 1999 | ||
| M13466917 | 4,053,946 | 2001 | ||
| M20996011 | 6,320,430 | 2003 | ||
| M24036583 | 7,235,733 | 2004 | ||
| M25964951 | 7,816,230 | 2005 | ||
| M30402457 | 9,152,052 | 2005 | ||
| M32582657 | 9,808,358 | 2006 | ||
| M43112609 | 12,978,189 | 2008 | ||
| M57885161 | 17,425,170 | 2013 | ||
| M74207281 | 22,338,618 | 2016 | ||
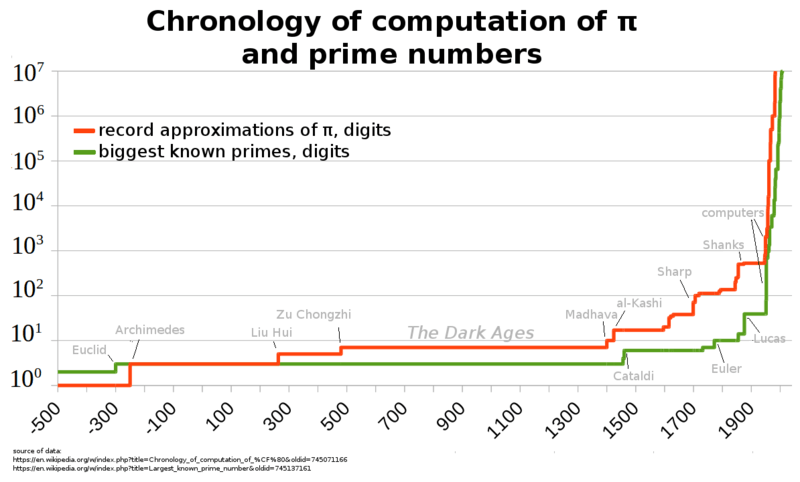
The twenty largest known prime numbers
| Rank | Prime number | Discovery date | Digits |
|---|---|---|---|
| 1 | 274207281 – 1 | 2016-01-07 | 22,338,618 |
| 2 | 257885161 – 1 | 2013-01-25 | 17,425,170 |
| 3 | 243112609 – 1 | 2008-08-23 | 12,978,189 |
| 4 | 242643801 – 1 | 2009-04-12 | 12,837,064 |
| 5 | 237156667 – 1 | 2008-09-06 | 11,185,272 |
| 6 | 232582657 – 1 | 2006-09-04 | 9,808,358 |
| 7 | 10223×231172165 + 1 | 2016-11-06 | 9,383,761 |
| 8 | 230402457 – 1 | 2005-12-15 | 9,152,052 |
| 9 | 225964951 – 1 | 2005-02-18 | 7,816,230 |
| 10 | 224036583 – 1 | 2004-05-15 | 7,235,733 |
| 11 | 220996011 – 1 | 2003-11-17 | 6,320,430 |
| 12 | 213466917 – 1 | 2001-11-14 | 4,053,946 |
| 13 | 19249×213018586 + 1 | 2007-03-26 | 3,918,990 |
| 14 | 3×211895718 − 1 | 2015-06-23[12] | 3,580,969 |
| 15 | 3×211731850 − 1 | 2015-03-13 | 3,531,640 |
| 16 | 3×211484018 − 1 | 2014-11-22 | 3,457,035 |
| 17 | 3×210829346 + 1 | 2014-01-14 | 3,259,959 |
| 18 | 475856524288 + 1 | 2012-08-08 | 2,976,633 |
| 19 | 356926524288 + 1 | 2012-06-20 | 2,911,151 |
| 20 | 341112524288 + 1 | 2012-06-20 | 2,900,832 |
GIMPS found the thirteen latest records on ordinary computers operated by participants around the world
See also
References
- ↑ Caldwell, Chris. "The largest known primes - Database Search Output". Prime Pages. Retrieved January 20, 2016.
- 1 2 Caldwell, Chris. "The Largest Known Prime by Year: A Brief History". Prime Pages. Retrieved January 20, 2016.
- ↑ The last non-Mersenne to be the largest known prime, was 391,581 ⋅ 2216,193 − 1; see also The Largest Known Prime by Year: A Brief History by Caldwell.
- ↑ "Mersenne Prime Number discovery - 274207281-1". Great Internet Mersenne Prime Search.
- 1 2 3 "Record 12-Million-Digit Prime Number Nets $100,000 Prize". Electronic Frontier Foundation. Electronic Frontier Foundation. October 14, 2009. Retrieved November 26, 2011.
- ↑ Electronic Frontier Foundation, Big Prime Nets Big Prize.
- ↑ "Best Inventions of 2008 - 29. The 46th Mersenne Prime". Time. Time Inc. October 29, 2008. Retrieved January 17, 2012.
- ↑ There is no mentioning among the ancient Egyptians of prime numbers, and they did not have any concept for prime numbers known today. In the Rhind papyrus (1650 BC) the Egyptian fraction expansions have fairly different forms for primes and composites, so it may be argued that they knew about prime numbers. "The Egyptians used ($) in the table above for the first primes r = 3, 5, 7, or 11 (also for r = 23). Here is another intriguing observation: That the Egyptians stopped the use of ($) at 11 suggests they understood (at least some parts of) Eratosthenes's Sieve 2000 years before Eratosthenes 'discovered' it." The Rhind 2/n Table [Retrieved 2012-11-11].
- ↑ Harris, H. S. The Reign of the Whirlwind, 1999 (p.252)
- ↑ Nicomachus' "Introduction to Arithmetic" translated by Martin Luther D'Ooge (p.52)
- ↑ "Euclid's Elements, Book IX, Proposition 36".
- ↑ "PrimeGrid's 321 Prime Search" (PDF). Retrieved October 12, 2016.
External links
- Press release about the largest known prime 274,207,281−1
- Press release about the former largest known prime 257,885,161−1
- Press release about the former largest known prime 243,112,609−1
- Press release about the former largest known prime 232,582,657−1